Materials that exhibit topological phases can be classified by their dimensionality, symmetries and topological invariants to form conductive-edge states with peculiar transport and spin properties. For example, the quantum Hall effect can arise in two-dimensional (2-D) electron systems subjected to a perpendicular magnetic field. When distinct characteristics of quantum Hall systems are compared with time-reversal symmetric (entropy conserved) topological insulators (TIs), they appear to rely on Coulomb interactions between electrons to induce a wealth of strongly correlated, topologically or symmetry-projected phases in a variety of experimental systems.

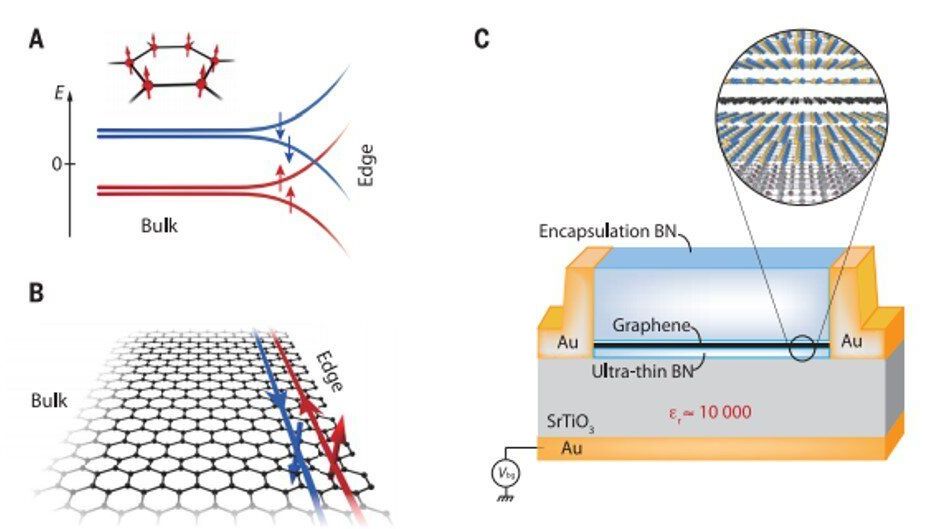
In a new report now on Science, Louis Veyrat and a research team in materials science, quantum optics and optoelectronics in France, China and Japan tuned the ground state of the graphene zeroth Landau level i.e. orbitals occupied by charged particles with discrete energy values. Using suitable screening of the Coulomb interaction with the high dielectric constant of a strontium titanate (SrTiO₃) substrate, they observed robust helical edge transport at magnetic fields as low as 1 Tesla, withstanding temperatures of up to 110 kelvin across micron-long distances. These versatile graphene platforms will have applications in spintronics and topological quantum computation.

Topological insulators (TIs), i.e., a material that behaves as an insulator in its interior but retains a conducting surface state, with zero Chern number have emerged as quantum Hall topological insulators (QHTIs) arising from many-body interacting Landau levels. They can be pictured as two independent copies of quantum Hall systems with opposite chirality, but the experimental system is at odds with the described scenario, where a strong insulating state is observed on increasing the perpendicular magnetic field in charge-neutral, high-mobility graphene devices.